Today's PUZZLE CLASSIC OF THE DAY
PREVIOUS 12 DAYS
One Protrusion, One Cutout
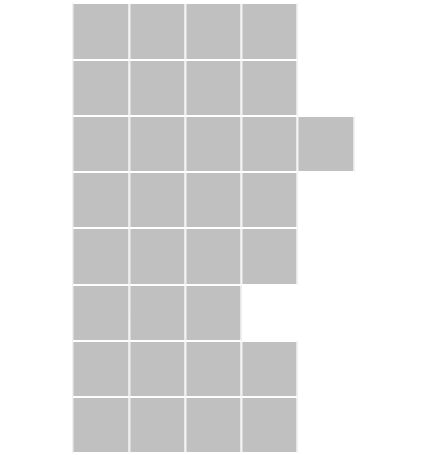
Cut this figure into 2 congruent parts (identical in area and shape, though they can be mirrored).
Cubed Calendar
This two-cube calendar numerically represents every day in a month. What are the four digits that are hidden from the view on the left cube, and the three on the right one?
Broken Digital Clock
Suppose you have a broken 12-hour digital clock that has its display turned on only when the number of hours is the same as the number of minutes - 08:08, 09:09, 10:10, and so on. What is the minimum time interval between such two “neighboring“ turnings?
Crossing Hour and Minute Hands
How many times each day do the minute and hour hands of a clock cross?
The Commute Challenge
If Walter walks to work in the morning and then takes the train back home in the evening, his round trip commute is 1h 30m. If he takes the train both ways, then the round trip commute is only 30 mins. How long would the round trip take him if by foot only?
The Volume of Which Cylinder is Bigger?
A Letter-sized sheet (8.5" x 11") is rolled into a cylinder along its long side (tall cylinder), while another - along its short side (short cylinder). Which of these two cylinders has the bigger volume?
A Unique 10-Digit Number
What makes this number unique? Drag the letters to answer.
Three Peasants, Eight Naan Breads
Two peasants, hungry on the way, decided to stop to eat. One had 5 naan breads with him, the other had 3. As soon as they were about to eat, a third peasant who had no food at all came up and asked them for permission to participate in the meal, promising to pay. They agreed. When all the naan breads were equally divided and eaten, the guest paid 8 rupees and left. How should the two peasants fairly share the money received?
Prune-Board into 4 Congruent Parts
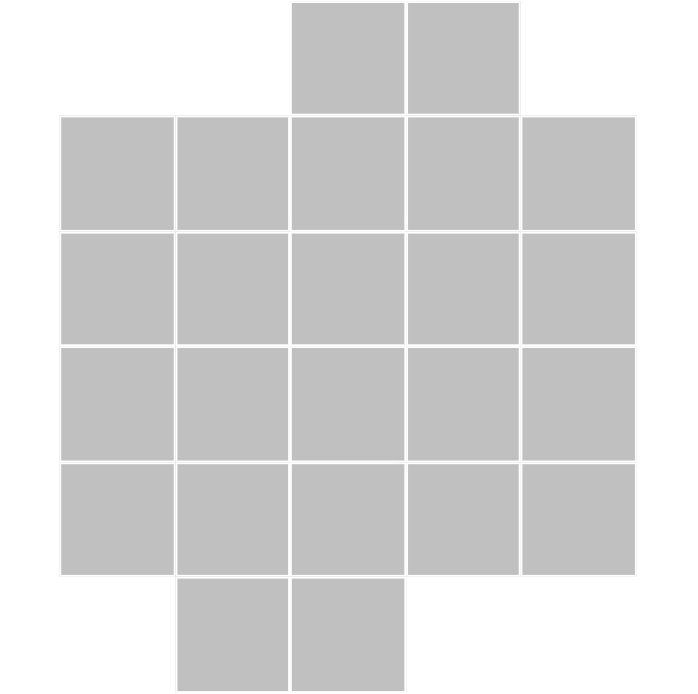
Cut this figure into 4 congruent parts (identical in area and shape, though they can be mirrored).
Shortest Catch
The ladybug wants to reach aphid as quickly as possible. Interestingly, the marked path is not the shortest route possible. How many shortest routes the ladybug can take from its current spot?
A Child's Age
A child's age (in full years) increased by 3 years gives a number which when square rooted results in a number obtained if the child's age is decreased by 3 years. Drag the number from the image to the circle(s) to indicate the child's age.
Adding the X
What number can be added to both 170 and 30 so that the resultant sums have a ratio of 3:1?